Chapter 1: Introduction to Maxwell's Equations

Maxwell's equations involve a significant amount of mathematics, but their implications are profound. Essentially, these equations reveal that light functions as an electromagnetic wave.

Gauss's Law

The version of Gauss's Law commonly taught in introductory physics courses states that the electric flux across a closed surface is proportional to the charge enclosed within that surface. The left side represents the electric flux, which is the integral of the electric field perpendicular to the surface. The right side quantifies the net charge enclosed by that surface, with ε₀ being a constant.

Essentially, this law asserts that electric charges produce electric fields, pointing away from positive charges and toward negative ones.

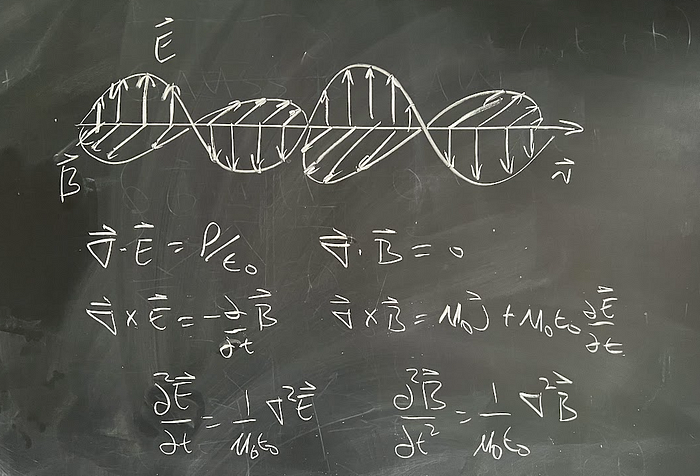
Gauss's Law for Magnetism

This law states that the magnetic flux through a closed surface is zero, indicating the absence of isolated magnetic charges, or magnetic monopoles. However, the existence of monopoles remains a theoretical possibility.

Faraday's Law

In addition to electric charges generating electric fields, a changing magnetic flux can also induce an electric field. The left side of the equation describes a closed line integral of the electric field, which is not zero, while the right side accounts for the magnetic flux through the area bounded by that path.

This leads us to categorize electric fields into two types: "Coulomb" fields from static charges and "curly" fields from changing magnetic flux.

Ampere-Maxwell Law

This equation indicates two mechanisms for creating a curly magnetic field: one through electric current and the other via changing electric flux. This law modifies Faraday's Law to include a term for electric current, and it indicates that magnetic fields can also be generated by electric currents.

Chapter 2: Differential Forms of Maxwell's Equations

In this section, we will explore the differential forms of Maxwell's equations, beginning with Gauss's Law. Here, we introduce the concept of charge density (ρ), which is charge per unit volume.

By employing the divergence theorem, we can relate surface area integrals to volume integrals, allowing us to express Gauss's Law in a new form.

For Gauss's Law for magnetism, since no magnetic monopoles exist, the magnetic charge density is zero, leading us to a simplified equation.

For Faraday's Law, we will utilize Stoke's Theorem to relate surface and line integrals. This theorem allows us to handle the time derivative more effectively, resulting in a refined expression.

The Wave Equation

Consider a scenario where one end of a string is shaken perpendicularly. This creates a displacement that travels along the string, leading to the wave equation, which describes the relationship between displacement, position, and time.

The wave speed, denoted by v, indicates how quickly the pulse moves along the string. For a more in-depth derivation of the wave equation, check out the following video:

The wave equation, expressed mathematically, shows how a function depends on both spatial and temporal variables. Interestingly, this wave equation can also be formulated in three dimensions for vector fields.

When no free charges or electric currents are present, Maxwell's equations can be simplified, leading to a fascinating result where both electric and magnetic fields satisfy their respective wave equations, each with a wave speed of 2.99 x 10⁸ m/s, which corresponds to the speed of light.

To verify that the magnetic field also follows a wave equation, we can apply a similar process starting from the Ampere-Maxwell equation and substituting using Faraday's Law.

In conclusion, Maxwell's equations not only define the behavior of electric and magnetic fields but also beautifully illustrate the wave nature of light.